Exercise 4
Q1 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 1
On a bargain counter, the shopkeeper puts labels on various goods showing their prices, Rs. P, where P is real numbers. Write a mathematical sentences for each of the following labels
(a) more than Rs. 7.50
(b) not less than Rs. 10
(c) not more than Rs. 22
(d) less than Rs. 11
Sol :
Since the price on label is Rs P, then
(a) more than Rs. 7.50 ⇒ P > 7.50
(b) not less than Rs. 10 ⇒ $P \not < 10$ or P ≥ 10
(c) not more than Rs. 22 ⇒ P ≯ 22 or P ≤ 10
(d) less than Rs. 11 ⇒ P < 11
Fill in the blanks
(a) A = {x : x ≥ -3 } = {……..}
(b) B = {x : x ≤ 1 } = {……..}
Sol :
You are given the following numbers : -2.6, 5.1, -3, 0.4, 1.2, -3.1, 4.7
It mean, you are given a replacement set as U = {-3.1, -3, -2.6, 0.4, 1.2, 4.7, 5.1}.
(a) Solution of A = {x : x ≥ -3 } = {-3, -2.6, 0.4, 1.2, 4.7, 5.1}
(b) Solution of B = {x : x ≤ 1 } = {-3.1, -3, -2.6, 0.4}
(a) $x +\frac{3}{2} > \frac{5}{2}$
(b) x – 4 = -3
(c) 2x – 5 ≥ 10
(d) $\frac{3y}{2} ≤ \frac{5}{2}$
(e) $4x^2 = 16$
Sol :
Since the replacement set is {-2, -1, +1, +2, +4, +5, +9}, then
(a) Solution set of $x + \frac{3}{2} > \frac{5}{2}$
(a) more than Rs. 7.50
(b) not less than Rs. 10
(c) not more than Rs. 22
(d) less than Rs. 11
Sol :
Since the price on label is Rs P, then
(a) more than Rs. 7.50 ⇒ P > 7.50
(b) not less than Rs. 10 ⇒ $P \not < 10$ or P ≥ 10
(c) not more than Rs. 22 ⇒ P ≯ 22 or P ≤ 10
(d) less than Rs. 11 ⇒ P < 11
Q2 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 2
You are given the following numbers : -2.6, 5.1, -3, 0.4, 1.2, -3.1, 4.7Fill in the blanks
(a) A = {x : x ≥ -3 } = {……..}
(b) B = {x : x ≤ 1 } = {……..}
Sol :
You are given the following numbers : -2.6, 5.1, -3, 0.4, 1.2, -3.1, 4.7
It mean, you are given a replacement set as U = {-3.1, -3, -2.6, 0.4, 1.2, 4.7, 5.1}.
(a) Solution of A = {x : x ≥ -3 } = {-3, -2.6, 0.4, 1.2, 4.7, 5.1}
(b) Solution of B = {x : x ≤ 1 } = {-3.1, -3, -2.6, 0.4}
Q3 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 3
If the replacement set is {-2, -1, +1, +2, +4, +5, +9}, what is the solution set of each of the following mathematical sentences.(a) $x +\frac{3}{2} > \frac{5}{2}$
(b) x – 4 = -3
(c) 2x – 5 ≥ 10
(d) $\frac{3y}{2} ≤ \frac{5}{2}$
(e) $4x^2 = 16$
Sol :
Since the replacement set is {-2, -1, +1, +2, +4, +5, +9}, then
(a) Solution set of $x + \frac{3}{2} > \frac{5}{2}$
⇒ $x > \frac{5}{2}– \frac{3}{2}$
⇒ x > 1 is {+2, +4, +5, +9}.
(b) Solution set of x – 4 = -3 ⇒ x = -3 + 4 ⇒ x = 1 is {+1}.
(c) Solution set of 2x – 5 ≥ 10 ⇒ 2x ≥ 15 ⇒ x ≥ 7.5 is {+9}.
(d) Solution set of $\frac{3y}{2} ≤ \frac{5}{2}$
(b) Solution set of x – 4 = -3 ⇒ x = -3 + 4 ⇒ x = 1 is {+1}.
(c) Solution set of 2x – 5 ≥ 10 ⇒ 2x ≥ 15 ⇒ x ≥ 7.5 is {+9}.
(d) Solution set of $\frac{3y}{2} ≤ \frac{5}{2}$
⇒ 3y ≤ 5
⇒ $y ≤ \frac{5}{3}$
⇒ y ≤ 1.66 is {-2, -1, +1}.
(e) Solution set of $4x^2 = 16 $
(e) Solution set of $4x^2 = 16 $
⇒ $x^2 = 4 $
⇒ x = +2 or -2 is {-2, +2}.
Q4 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 4
List the solution set of 30 – 4(2x – 1) < 30, given that x is a positive integer.Sol :
Since the replacement set is a positive integer, then
Solution set of
⇒ 30 – 4(2x – 1) < 30
⇒ -4(2x – 1) < 30 – 30
⇒ -4(2x – 1) < 0
⇒ (2x – 1) > 0
⇒ $x > \frac{1}{2}$
⇒ x > 0.5
is x = {1, 2, 3, 4, 5,…}
Q5 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 5
If the replacement set is {0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10}, what is the solution set of each of the following mathematical sentences.(a) $x + \frac{4}{3} = \frac{7}{3}$
(b) 2x + 1 < 3
(c) x – 6 > 10 – 6
(d) x + 5 = 20
(e) 2x + 3 ≥ 17
Sol :
Since the replacement set is {0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10}, then
(a) Solution set of $x + \frac{4}{3} = \frac{7}{3} $
⇒ $x = \frac{3}{3}$
⇒ x = 1 is {1}.
(b) Solution set of 2x + 1 < 3 ⇒ 2x < 2 ⇒ x < 1 is {0}.
(c) Solution set of x – 6 > 10 – 6 ⇒ x > 10 is φ.
(d) Solution set of x + 5 = 20 ⇒ x = 15 is φ.
(e) Solution set of 2x + 3 ≥ 17 ⇒ 2x ≥ 14 ⇒ x ≥ 7 is {7, 8, 9}.
(b) Find the truth set of the inequality x > y + 2, where (x, y) ∈ {(1, 2), (2, 3), (5, 1), (7, 3), (5, 6)}
Sol :
(a) x ∈ {2, 4, 6, 9} and y ∈ {4, 6, 18, 27, 54}.
(x, y) = x is a factor of y and x < y.
2 is a factor of 4, 6, 18, 54 and 2 < 4, 2 < 6, 2 < 18, 2 < 54, then (x, y) forms the ordered pairs as (2, 4), (2, 6), (2, 18), (2, 54).
4 is a factor of 4 but 4 = 4, then (4, 4) is not in (x, y).
6 is a factor of 18, 54 and 6 < 18, 6 < 54, then (x, y) forms the ordered pairs as (6, 18), (6, 54).
9 is a factor of 18, 27, 54 and 9 < 18, 9 < 27, 9 < 54 then (x, y) forms the ordered pairs as (9, 18), (9, 27), (9, 54).
Thus, the solution set of (x, y) is {(2, 4), (2, 6), (2, 18), (2, 54), (6, 18), (6, 54), (9, 18), (9, 27), (9, 54)}.
(b) Given inequality is x > y + 2. It means x is greater than y + 2.
In {(1, 2), (2, 3), (5, 1), (7, 3), (5, 6)}, we see that (5, 1) and (7, 3) are two pairs which satisfy the given inequality.
Thus, the solution set of (x, y) is {(5, 1), (7, 3)}.
(i) $\frac{5}{x}> 7$ ; {1, 2}
(ii) $\frac{5}{x}> 2$ ; {1, 2, 3, 4, 5, 6}
(iii) $x^2 = 9 $; {-3, -2, -1, 1, 2, 3}
(iv) $x + \frac{1}{x}$= 2 ; {0, 1, 2, 3}
(v) $3x^2 < 2x$ ; {-4, -3, -2, -1, 0, 1, 2, 3, 4}
(vi) 2(x – 3) < 1 ; {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}
Sol :
(i) Given replacement set is {1, 2}.
Now given inequality is $\frac{5}{x}> 7 $
⇒ $x < \frac{5}{7} $
⇒ x < 0.71
Thus, the solution set of the given inequality is x ∈ φ.
(ii) Given replacement set is {1, 2, 3, 4, 5, 6}.
Now given inequality is $\frac{5}{x}> 2 $
⇒ $x < \frac{5}{2}$
⇒ x < 2.5
Thus, the solution set of the given inequality is x ∈ {1, 2}.
(iii) Given replacement set is {-3, -2, -1, 1, 2, 3}.
Now given equation is $x^2 = 9 $
⇒ x = 3 or -3.
Thus, the solution set of the given equation is x ∈ {-3, 3}.
(iv) Given replacement set is {0, 1, 2, 3}.
Now given equation is
$x + \frac{1}{x} = 2$ ⇒ $(x^2 + 1) = 2x $
⇒$(x2 -2x + 1) = 0 $
⇒ (x – 1)2 = 0
⇒ (x – 1) = 0
⇒ x = 1.
Thus, the solution set of the given equation is x ∈ {1}.
(v) Given replacement set is {-4, -3, -2, -1, 0, 1, 2, 3, 4}.
Now given inequality is $3x^2 < 2x $
⇒$ 3x^2 – 2x < 0 $
⇒ x(3x – 2) < 0
⇒ $0 < x < \frac{2}{3} $
⇒ 0 < x < 0.66
Thus, the solution set of the given inequality is x ∈ φ.
(vi) Given replacement set is {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}.
Now given inequality is 2(x – 3) < 1 ⇒ x – 3 < 0.5 ⇒ x < 3.5.
Thus, the solution set of the given inequality is x ∈ {1, 2, 3}.
(b) Solution set of 2x + 1 < 3 ⇒ 2x < 2 ⇒ x < 1 is {0}.
(c) Solution set of x – 6 > 10 – 6 ⇒ x > 10 is φ.
(d) Solution set of x + 5 = 20 ⇒ x = 15 is φ.
(e) Solution set of 2x + 3 ≥ 17 ⇒ 2x ≥ 14 ⇒ x ≥ 7 is {7, 8, 9}.
Q6 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 6
(a) x ∈ {2, 4, 6, 9} and y ∈ {4, 6, 18, 27, 54}. Form all ordered pairs (x, y) such that x is a factor of y and x < y.(b) Find the truth set of the inequality x > y + 2, where (x, y) ∈ {(1, 2), (2, 3), (5, 1), (7, 3), (5, 6)}
Sol :
(a) x ∈ {2, 4, 6, 9} and y ∈ {4, 6, 18, 27, 54}.
(x, y) = x is a factor of y and x < y.
2 is a factor of 4, 6, 18, 54 and 2 < 4, 2 < 6, 2 < 18, 2 < 54, then (x, y) forms the ordered pairs as (2, 4), (2, 6), (2, 18), (2, 54).
4 is a factor of 4 but 4 = 4, then (4, 4) is not in (x, y).
6 is a factor of 18, 54 and 6 < 18, 6 < 54, then (x, y) forms the ordered pairs as (6, 18), (6, 54).
9 is a factor of 18, 27, 54 and 9 < 18, 9 < 27, 9 < 54 then (x, y) forms the ordered pairs as (9, 18), (9, 27), (9, 54).
Thus, the solution set of (x, y) is {(2, 4), (2, 6), (2, 18), (2, 54), (6, 18), (6, 54), (9, 18), (9, 27), (9, 54)}.
(b) Given inequality is x > y + 2. It means x is greater than y + 2.
In {(1, 2), (2, 3), (5, 1), (7, 3), (5, 6)}, we see that (5, 1) and (7, 3) are two pairs which satisfy the given inequality.
Thus, the solution set of (x, y) is {(5, 1), (7, 3)}.
Q7 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 7
Find out the truth set of the following open sentences replacement sets are given(i) $\frac{5}{x}> 7$ ; {1, 2}
(ii) $\frac{5}{x}> 2$ ; {1, 2, 3, 4, 5, 6}
(iii) $x^2 = 9 $; {-3, -2, -1, 1, 2, 3}
(iv) $x + \frac{1}{x}$= 2 ; {0, 1, 2, 3}
(v) $3x^2 < 2x$ ; {-4, -3, -2, -1, 0, 1, 2, 3, 4}
(vi) 2(x – 3) < 1 ; {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}
Sol :
(i) Given replacement set is {1, 2}.
Now given inequality is $\frac{5}{x}> 7 $
⇒ $x < \frac{5}{7} $
⇒ x < 0.71
Thus, the solution set of the given inequality is x ∈ φ.
(ii) Given replacement set is {1, 2, 3, 4, 5, 6}.
Now given inequality is $\frac{5}{x}> 2 $
⇒ $x < \frac{5}{2}$
⇒ x < 2.5
Thus, the solution set of the given inequality is x ∈ {1, 2}.
(iii) Given replacement set is {-3, -2, -1, 1, 2, 3}.
Now given equation is $x^2 = 9 $
⇒ x = 3 or -3.
Thus, the solution set of the given equation is x ∈ {-3, 3}.
(iv) Given replacement set is {0, 1, 2, 3}.
Now given equation is
$x + \frac{1}{x} = 2$ ⇒ $(x^2 + 1) = 2x $
⇒$(x2 -2x + 1) = 0 $
⇒ (x – 1)2 = 0
⇒ (x – 1) = 0
⇒ x = 1.
Thus, the solution set of the given equation is x ∈ {1}.
(v) Given replacement set is {-4, -3, -2, -1, 0, 1, 2, 3, 4}.
Now given inequality is $3x^2 < 2x $
⇒$ 3x^2 – 2x < 0 $
⇒ x(3x – 2) < 0
⇒ $0 < x < \frac{2}{3} $
⇒ 0 < x < 0.66
Thus, the solution set of the given inequality is x ∈ φ.
(vi) Given replacement set is {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}.
Now given inequality is 2(x – 3) < 1 ⇒ x – 3 < 0.5 ⇒ x < 3.5.
Thus, the solution set of the given inequality is x ∈ {1, 2, 3}.
Q8 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 8
Let x, x+1, x+2 be the lengths of the three sides of a triangle.
(i) Write down the three inequations in x, each of which represents the given statement.
(ii) List the set of possible values of x which satisfy all the three inequations obtained in your answer to part (i) above, given that x is an integer.
Sol :
The lengths of the three sides of a triangle are x, x+1, x+2.
(i)
Since the sum of the lengths of any two sides of a triangle is always greater than the length of its third side, we have three inequality as below.
x + (x + 1) > (x + 2) ⇒ 2x + 1 > x + 2 ⇒ x > 1 …(1)
x + (x + 2) > (x + 1) ⇒ 2x + 2 > x + 1 ⇒ x > -1 …(2)
(x + 1) + (x + 2) > x ⇒ 2x + 3 > x ⇒ x > -3 …(3)
On combining (1), (2), (3), we get x > 1.
(ii) Since replacement set of x is an integer, means x ∈ {…, -3, -2, -1, 0, 1, 2, 3, …}.
The solution set of x > 1 is x ∈ { 2, 3, 4, …}.
Q9 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 9
(a) If x + 10 = y + 14, then x > y
(b) |-4| – 4 = 8
(c) If 10 – x > 3, then x < 7
(d) If p = q + 2, then p > q
(e) If a and b are two negative integers such that a < b, then $\frac{1}{a} < \frac{1}{b}$
(f) 3 ∈ {x : 3x – 2 ≥ 5}
Sol :
(a) x + 10 = y + 14
⇒ x – y = 14 – 10
⇒ x – y = 4
⇒ x – y > 0
⇒ x > y.
Thus, the given statement “If x + 10 = y + 14, then x > y” is TRUE.
(b) |-4| – 4 = 4 – 4 = 0
Thus, the given statement “|-4| – 4 = 8” is FALSE.
(c) 10 – x > 3
Thus, the given statement “If x + 10 = y + 14, then x > y” is TRUE.
(b) |-4| – 4 = 4 – 4 = 0
Thus, the given statement “|-4| – 4 = 8” is FALSE.
(c) 10 – x > 3
⇒ 10 – 3 > x
⇒ 7 > x
⇒ x > 7
Thus, the given statement “If 10 – x > 3, then x < 7” is TRUE.
(d) p = q + 2
Thus, the given statement “If 10 – x > 3, then x < 7” is TRUE.
(d) p = q + 2
⇒ p – q = 2
⇒ p – q > 0
⇒ p > q
Thus, the given statement “If p = q + 2, then p > q” is TRUE.
(e) a < b
Thus, the given statement “If p = q + 2, then p > q” is TRUE.
(e) a < b
⇒ $\frac{1}{a} > \frac{1}{b}$
Thus, the given statement “If a and b are two negative integers such that a < b, then $\frac{1}{a} < \frac{1}{b}$” is FALSE.
(f) 3x – 2 ≥ 5
Thus, the given statement “If a and b are two negative integers such that a < b, then $\frac{1}{a} < \frac{1}{b}$” is FALSE.
(f) 3x – 2 ≥ 5
⇒ 3x ≥ 7
⇒ $x ≥ \frac{7}{3}$
⇒ x ≥ 2.3
Thus, the given statement “3 ∈ {x : 3x – 2 ≥ 5}” is TRUE.
Thus, the given statement “3 ∈ {x : 3x – 2 ≥ 5}” is TRUE.
Q10 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 10
Sol :
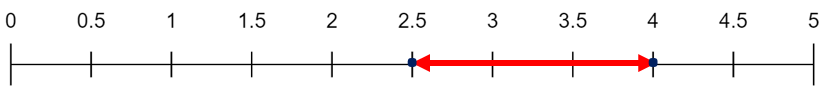
⇒ 2 ≤ 2p – 3 ≤ 5
⇒ 5 ≤ 2p ≤ 8
⇒ $\frac{5}{2} ≤ p ≤ 4 $
⇒ 2.5 ≤ p ≤ 4
The solution on the number line is as below.
If x is a negative integer, find the solution set of $\frac{2}{3} + \frac{(x + 1)}{3}> 0$.
Sol :
⇒ $\frac{2}{3} + \frac{(x + 1)}{3} > 0$
⇒ (x + 1)/3 > -2/3
⇒ x + 1 > -2
⇒ x > -3 …(1)
Since the replacement set of x is negative integer, it means x ∈ {…, -5, -4, -3, -2, -1}.
From (1), x ∈ {-2, -1}.
Sol :
(i)
From the graph, we see that the shaded arrow is left of -2 (included) and all the real numbers are taken up to -2.
Thus, required mathematical sentence is {x : x ≤ -2 and x ∈ R}.
(ii)
From the graph, we see that the shaded arrow is left of 4 (included) and all the real numbers are taken up to 4.
Thus, required mathematical sentence is {x : x ≤ 4 and x ∈ R}.
(iii)
From the graph, we see that the solid circles are at 4 and 5 and only natural numbers are taken.
Thus, required mathematical sentence is {x : 4 ≤ x ≤ 5 and x ∈ N}.
(iv)
From the graph, we see that the solid circles are at 1, 3 and 5 and only natural numbers are taken.
Thus, required mathematical sentence is {x : 1 ≤ x ≤ 5 and x ∈ N}.
(v)
From the graph, we see that the shaded arrow is right of -2 (excluded) and all the real numbers are ahead of -2.
Thus, required mathematical sentence is {x : x > -2 and x ∈ R}.
(i) If 2 – x < 0, then x > 2.
(ii) The graph of the inequations y ≤ 2x includes the origin.
Sol :
(i) 2 – x < 0 ⇒ 2 < x.
Thus, the given statement is TRUE.
(ii) On putting x = 0 and y = 0 in y ≤ 2x, we get that 0 = 0, which is true.
Thus, the given statement is TRUE.
⇒ 5 ≤ 2p ≤ 8
⇒ $\frac{5}{2} ≤ p ≤ 4 $
⇒ 2.5 ≤ p ≤ 4
The solution on the number line is as below.
Q11 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 11
Sol :
⇒ $\frac{2}{3} + \frac{(x + 1)}{3} > 0$
⇒ (x + 1)/3 > -2/3
⇒ x + 1 > -2
⇒ x > -3 …(1)
Since the replacement set of x is negative integer, it means x ∈ {…, -5, -4, -3, -2, -1}.
From (1), x ∈ {-2, -1}.
Q12 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 12
Write open mathematical sentences, using x for the variable whose graphs would be
Sol :
(i)
From the graph, we see that the shaded arrow is left of -2 (included) and all the real numbers are taken up to -2.
Thus, required mathematical sentence is {x : x ≤ -2 and x ∈ R}.
(ii)
From the graph, we see that the shaded arrow is left of 4 (included) and all the real numbers are taken up to 4.
Thus, required mathematical sentence is {x : x ≤ 4 and x ∈ R}.
(iii)
From the graph, we see that the solid circles are at 4 and 5 and only natural numbers are taken.
Thus, required mathematical sentence is {x : 4 ≤ x ≤ 5 and x ∈ N}.
(iv)
From the graph, we see that the solid circles are at 1, 3 and 5 and only natural numbers are taken.
Thus, required mathematical sentence is {x : 1 ≤ x ≤ 5 and x ∈ N}.
(v)
From the graph, we see that the shaded arrow is right of -2 (excluded) and all the real numbers are ahead of -2.
Thus, required mathematical sentence is {x : x > -2 and x ∈ R}.
Q13 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 13
Answer TRUE or FALSE:(i) If 2 – x < 0, then x > 2.
(ii) The graph of the inequations y ≤ 2x includes the origin.
Sol :
(i) 2 – x < 0 ⇒ 2 < x.
Thus, the given statement is TRUE.
(ii) On putting x = 0 and y = 0 in y ≤ 2x, we get that 0 = 0, which is true.
Thus, the given statement is TRUE.
Q14 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 14
(i) the smallest value of x when x is real number.
(ii) the smallest values of x when x is an integer.
Sol :
⇒ 25 – 4x ≤ 16
⇒ 25 – 16 ≤ 4x
⇒ 9 ≤ 4x
⇒$ x ≥ \frac{9}{4}$
(i) Thus, the smallest value of x is $\frac{9}{4} = 2 \frac{1}{4}$.
(ii) The smallest integer greater than $x ≥ \frac{9}{4}$ is 3.
Given x ∈ {1, 2, 3, 4, 5, 6, 7, 9}, find the values of x for which -3 < 2x – 1< x + 4
Sol :
Given replacement set is x ∈ {1, 2, 3, 4, 5, 6, 7, 9}.
Given inequation is -3 < 2x – 1 < x + 4.
On solving -3 < 2x – 1 ⇒ 2x > -3 + 1 ⇒ 2x > -2 ⇒ x > -1 … (1)
On solving 2x – 1 < x + 4 ⇒ 2x – x < 1 + 4 ⇒ x < 5 …(2)
From (1) and (2), we get -1 < x < 5.
Thus, the solution set of the given inequation is x ∈ {1, 2, 3, 4}.
(i) Thus, the smallest value of x is $\frac{9}{4} = 2 \frac{1}{4}$.
(ii) The smallest integer greater than $x ≥ \frac{9}{4}$ is 3.
Q15 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 15
Sol :
Given replacement set is x ∈ {1, 2, 3, 4, 5, 6, 7, 9}.
Given inequation is -3 < 2x – 1 < x + 4.
On solving -3 < 2x – 1 ⇒ 2x > -3 + 1 ⇒ 2x > -2 ⇒ x > -1 … (1)
On solving 2x – 1 < x + 4 ⇒ 2x – x < 1 + 4 ⇒ x < 5 …(2)
From (1) and (2), we get -1 < x < 5.
Thus, the solution set of the given inequation is x ∈ {1, 2, 3, 4}.
Q16 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 16
Solve the inequality 2x – 10 < 3x – 15Sol :
Given inequality is 2x – 10 < 3x – 15
⇒ 2x – 3x < 10 – 15
⇒ -x < -5
⇒ x > 5
Q17 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 17
Sol :
Given inequation is 3 – 2x ≥ x – 12
⇒ -x – 2x ≥ -3 – 12
⇒ -3x ≥ -15
⇒ x ≤ 5
Since the replacement of x is natural number, then the solution set of the given inequation is
x ∈ {1, 2, 3, 4, 5}.
Q18 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 18
Sol :
Given inequation is -1 < 3 – 2x ≤ 7
From -1 < 3 – 2x,
⇒ -1 < 3 – 2x
⇒ -1 – 3 < – 2x
⇒ -4 < -2x
⇒ 2x < 4
⇒ x < 2 …(1)
and 3 – 2x ≤ 7
⇒ 3 – 7 ≤ 2x ⇒ -4 ≤ 2x ⇒ -2 ≤ x …(2)
On combining (1) and (2), we get -2 ≤ x < 2.
The graph of this inequation is

Q19 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 19
$-2 \frac{2}{3} \leq x+\frac{1}{3}<3 \frac{1}{3}, x \in R$
Sol :
Given inequation is
$-2 \frac{2}{3} \leq x+\frac{1}{3}<3 \frac{1}{3}, x \in R$
On simplifying, we get
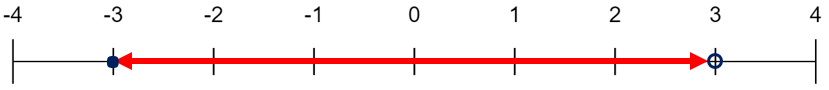
$\Rightarrow-\frac{8}{3} \leq x+\frac{1}{3}<\frac{10}{3}$
$\Rightarrow-\frac{8}{3}-\frac{1}{3} \leq x+\frac{1}{3}-\frac{1}{3}<\frac{10}{3}-\frac{1}{3}$
$\Rightarrow-\frac{9}{3} \leq x<\frac{9}{3}$
$\Rightarrow-3 \leq x<3$
Now the graph of these values of x is as below.
Q20 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 20
(a) $6 \geq 2-x, \frac{x}{3}+2<3 ; x \in R$
(b) $\frac{x}{2}<\frac{6-x}{4}, \frac{2-x}{6}<\frac{7-x}{9} ; x \in R$
Sol :
(a) Given inequation is
$6 \geq 2-x, \frac{x}{3}+2<3 ; x \in R$
On solving first inequation, we get
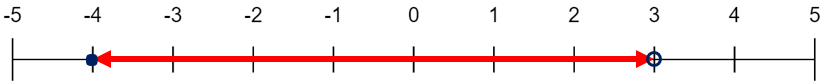
⇒ 6 ≥ 2 – x
⇒ x ≥ 2 – 6
⇒ x ≥ -4 … (1)
On solving second inequation, we get $=\frac{x}{3} + 2 < 3$
⇒ $\frac{x}{3} < 3 – 2$
⇒$\frac{x}{3} < 1$
⇒ x < 3 … (2)
On combining (1) and (2), we get -4 ≤ x < 3.
The graph of this inequation is
$\frac{x}{2}<\frac{6-x}{4}, \frac{2-x}{6}<\frac{7-x}{9} ; x \in R$
On solving first inequation, we get
⇒ $\frac{x}{2} < \frac{(6 – x)}{4}$
⇒ 2x < 6 – x
⇒ 3x < 6
⇒ x < 2… (1)
On solving second inequation, we get
⇒ $\fraC{(2 – x)}{6} < \frac{(7 – x)}{9}$
On solving second inequation, we get
⇒ $\fraC{(2 – x)}{6} < \frac{(7 – x)}{9}$
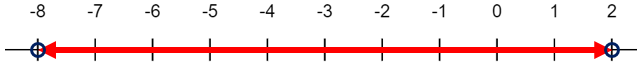
⇒ 3(2 – x) < 2(7 – x)
⇒ 6 – 3x < 14 – 2x
⇒ x > -8 … (2)
On combining (1) and (2), we get -8 < x < 2.
The graph of this inequation is
Given inequation is
$-\dfrac{1}{3}\leq \dfrac{x}{2}-1\dfrac{1}{3}<\dfrac{1}{6}; x\in R$
On simplifying,we get
$\begin{aligned}On combining (1) and (2), we get -8 < x < 2.
The graph of this inequation is
Q21 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 21
Find the range of values of x which satisfy
$-\dfrac{1}{3}\leq \dfrac{x}{2}-1\dfrac{1}{3}<\dfrac{1}{6}; x\in R$
Graph these values of x on real number line.
Sol :Given inequation is
$-\dfrac{1}{3}\leq \dfrac{x}{2}-1\dfrac{1}{3}<\dfrac{1}{6}; x\in R$
On simplifying,we get
&\Rightarrow-\frac{1}{3} \leq \frac{x}{2}-\frac{4}{3}<\frac{1}{6} \\
&\Rightarrow-\frac{1}{3}+\frac{4}{3} \leq \frac{x}{2}<\frac{1}{6}+\frac{4}{3} \\
&\Rightarrow 1 \leq \frac{x}{2}<\frac{3}{2} \\
&\Rightarrow 2 \leq x<3
\end{aligned}$
Graph of these values of x on number line is as below. 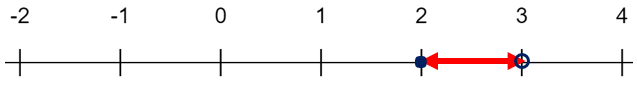
Q22 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 22
Sol :
Graphing the given inequations x > 3 and -2 ≤ x < 5 on same number line, we get 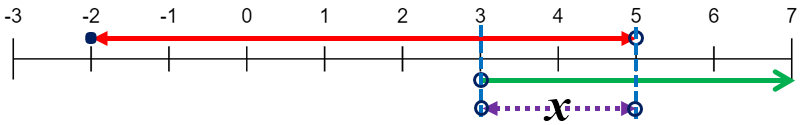
Q23 | Ex-4 | Class 8 | S.Chand | Mathematics | Chapter 4 | Linear Inequations | myhelper
OPEN IN YOUTUBE
Question 23
Solve and graph the solution set of 3x – 4 > 11 or 5 – 2x ≥ 7; x ∈ R.Sol :
On solving the first inequation, we get
⇒ 3x – 4 > 11
⇒ 3x > 15
⇒ x > 5 …(1)
On solving the second inequation, we get
On solving the second inequation, we get
⇒ 5 – 2x ≥ 7
⇒ – 2x ≥ 2
⇒ x ≤ 1 …(2)
On combining (1) and (2), we get x ≤ 1 or x > 5.
On combining (1) and (2), we get x ≤ 1 or x > 5.
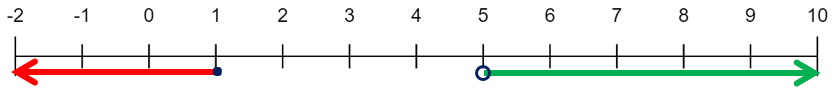
No comments:
Post a Comment